Inverse derivative is a fundamental concept in calculus that plays a crucial role in understanding the relationship between functions and their rates of change. In this article, we will explore the inverse derivative, its significance, applications, and much more. Whether you are a student, educator, or simply curious about mathematics, this guide will provide you with valuable insights into this complex yet fascinating topic.
The concept of derivatives is essential in calculus; however, the idea of an inverse derivative often raises questions. What does it mean to find an inverse of a derivative? How does it affect the original function? These are some of the questions we will address in this article. We will dive deep into the mathematical principles, explore relevant examples, and provide practical applications to enhance your understanding.
In addition to the theoretical aspects, we will also discuss the role of inverse derivatives in real-world scenarios, including physics, engineering, and economics. By the end of this article, you will have a comprehensive understanding of inverse derivatives and how they can be applied in various fields. Let’s get started!
Table of Contents
- What is Inverse Derivative?
- Mathematical Principles of Inverse Derivative
- Applications of Inverse Derivative
- Examples of Inverse Derivative
- The Inverse Derivative Theorem
- Common Misconceptions about Inverse Derivatives
- Graphical Representation of Inverse Derivatives
- Conclusion
What is Inverse Derivative?
Inverse derivative refers to the process of finding the original function from its derivative. In simpler terms, if we have a function \( f(x) \), its derivative is represented as \( f'(x) \). The inverse derivative seeks to determine \( f(x) \) when given \( f'(x) \). This concept is closely related to integration, where the integral of a function can be seen as finding its inverse derivative.
Definition and Explanation
To understand inverse derivatives, we must first grasp what a derivative is. A derivative represents the rate of change of a function with respect to its variable. Conversely, the inverse derivative allows us to understand how the original function behaves based on its rate of change.
Importance in Calculus
Inverse derivatives are significant in calculus as they connect differentiation and integration. The Fundamental Theorem of Calculus states that differentiation and integration are inverse processes. This relationship is vital for solving complex problems in mathematics and its applications in science and engineering.
Mathematical Principles of Inverse Derivative
To delve deeper into the mathematical principles behind inverse derivatives, we need to explore several key concepts, including the chain rule, implicit differentiation, and the relationship between functions and their inverses.
Chain Rule and Inverse Derivatives
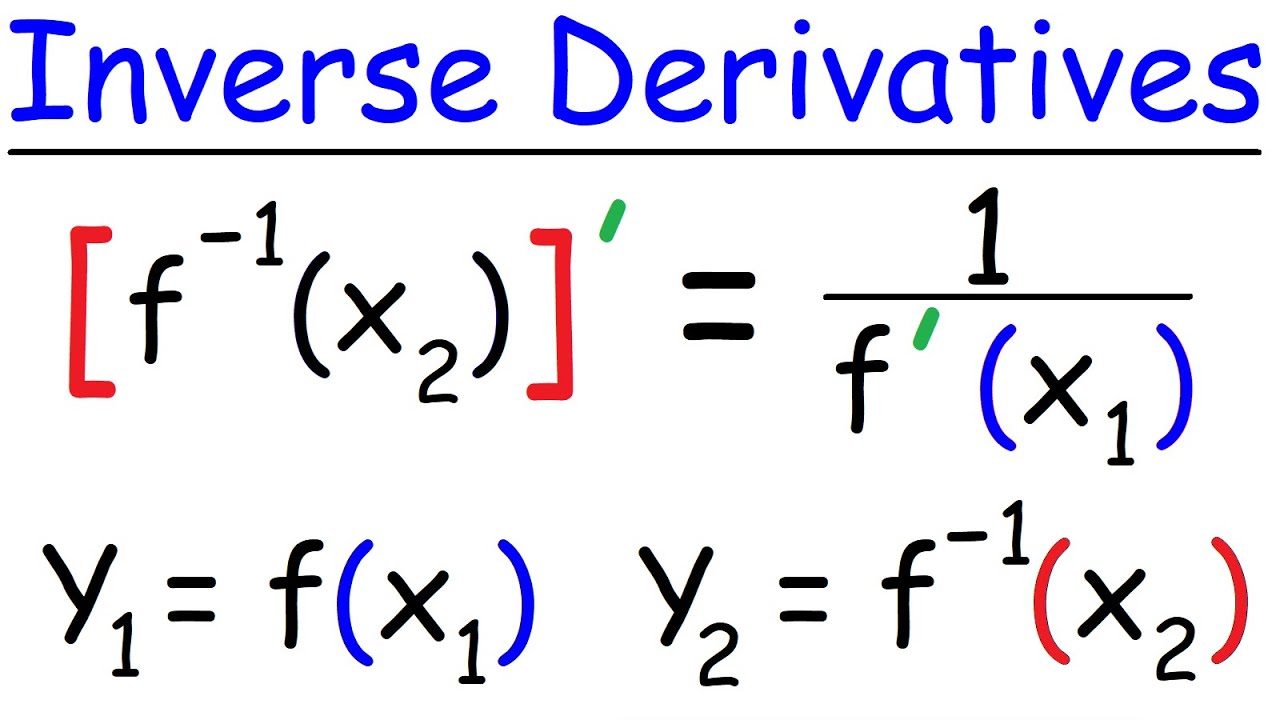
The chain rule is a fundamental theorem in calculus that allows us to differentiate composite functions. When dealing with inverse derivatives, the chain rule becomes crucial. If we have a function \( y = f(x) \), its inverse can be represented as \( x = f^{-1}(y) \). The derivative of the inverse function can be calculated using the chain rule as follows:
- If \( y = f(x) \), then \( \frac{dy}{dx} = f'(x) \).
- For the inverse, \( \frac{dx}{dy} = \frac{1}{f'(x)} \) where \( x = f^{-1}(y) \).
Implicit Differentiation
Implicit differentiation is another critical concept that aids in finding inverse derivatives. When the relationship between \( x \) and \( y \) is not explicitly defined, we can differentiate both sides concerning one variable while treating the other as a function of the first. This technique is particularly useful in solving problems involving inverse functions.
Applications of Inverse Derivative
Inverse derivatives have numerous applications across various fields, including physics, engineering, economics, and data science. Understanding how these derivatives can be applied provides valuable insights into real-world problems.
Physics and Engineering
In physics, inverse derivatives are often used to analyze motion. For example, if we know the velocity of an object as a function of time, we can find its position by calculating the inverse derivative. This application is fundamental in kinematics and dynamics.
Economics and Finance
In economics, inverse derivatives can help in understanding the relationship between supply and demand. By analyzing how changes in price affect quantity demanded or supplied, economists can make informed predictions about market behavior.
Examples of Inverse Derivative
To solidify your understanding of inverse derivatives, let’s look at a few examples that illustrate the concept in action.
Example 1: Basic Inverse Derivative
Consider the function \( f(x) = x^2 \). The derivative is \( f'(x) = 2x \). To find the inverse derivative, we can derive the original function:
- Let \( y = 2x \).
- Solving for \( x \) gives \( x = \frac{y}{2} \).
- Thus, the inverse function is \( f^{-1}(y) = \sqrt{y} \).
Example 2: Application in Motion
Let’s say the velocity of an object is given by \( v(t) = 3t^2 \). To find the position function, we calculate the inverse derivative:
- Integrate \( v(t) \) to find \( s(t) = \int 3t^2 dt = t^3 + C \).
- Thus, the position function is \( s(t) = t^3 + C \), where \( C \) is the constant of integration.
The Inverse Derivative Theorem
The Inverse Derivative Theorem provides a formal framework for understanding the relationship between a function and its inverse. It states that if \( f \) is continuous and differentiable in an interval, and \( f' \) is non-zero, then the inverse \( f^{-1} \) exists and is also differentiable.
Conditions for the Inverse Derivative Theorem
- Function \( f \) must be one-to-one.
- Derivative \( f' \) must be non-zero in the interval.
Significance of the Theorem
This theorem is significant because it assures us that under certain conditions, we can confidently find the inverse derivative and utilize it in various applications without ambiguity.
Common Misconceptions about Inverse Derivatives
While inverse derivatives are crucial in calculus, several misconceptions can lead to confusion. Here are some common misunderstandings:
Misconception 1: Inverse Derivative is the Same as Derivative
Many students mistakenly believe that the inverse derivative is the same as the derivative. In reality, they serve different purposes and represent different mathematical concepts.
Misconception 2: Inverse Derivatives Always Exist
Another common misconception is that inverse derivatives always exist for any function. This is not true; the existence of an inverse derivative depends on the properties of the function, particularly its continuity and differentiability.
Graphical Representation of Inverse Derivatives
Visualizing the concept of inverse derivatives can greatly enhance understanding. The graph of a function and its inverse can provide insights into their relationships.
Graph of a Function vs. Its Inverse
The graph of a function \( f(x) \) and its inverse \( f^{-1}(x) \) are reflections of each other across the line \( y = x \). This symmetry illustrates the relationship between the two functions and can help clarify the process of finding inverse derivatives.
Example of Graphical Representation
For instance, consider the function \( f(x) = x^2 \) (for \( x \geq 0 \)). Its inverse function is \( f^{-1}(x) = \sqrt{x} \). Plotting both functions on the same graph shows how they mirror each other across the line \( y = x \).
Kristy Mcnichol